(This post originally started as notes to myself, placed here so that I
would not lose or forget them.)
The Digital Down Converter (DDC) is a basic block of FPGA-implemented SDR radios. Per Wikipedia:
Note that, for demonstration purposes, the equations assume a simple cosine as the input signal.
This post will look at the math involved in the Mixer. I will leave the low-pass filter and sample-rate conversion for later posts.
First, some basics...
Basic Down-Conversion Mixing:
Historically, down-conversion has been done in the analog domain with a "real" oscillator signal (e.g. cos(ωot)). Let's first look at this type of basic mixer before tackling mixing in the Digital (and Complex) domain.
For demonstration purposes, I'll simplify the receive-signal to be a just simple cosine signal at a single frequency: cos(ωit).
This mixer's math is straightforward. Let the output of the mixer be y(t).
But this basic form of mixing has a problem if one would like to down-convert
an incoming RF signal directly to base-band: both the desired signal and its image will appear in the base-band spectrum (which, for the typical shortwave
receiver, is the audio spectrum we want to hear).
This type of conversion is known as "Direct Conversion." For an example, see Rick Campbell's R1 receiver.
Visually, here's how the frequencies shift, shown as a single-sided spectrum (which one would see if one did a "Trigonometric" Fourier Transform on the RF input. Refer to equation 27 in this paper by Robert Marcus for more detail)::
Note the overlap of the two signals down at base-band...our "desired" signal would be interfered with by any signal at its "image" on the other-side of the oscillator's frequency!
And if we were to consider the Mixer's input to be a band of frequencies:
You can see the spectrum fold-back with the result being the desired signal and its undesired image occupying the same base-band frequencies. A single-sided spectrum graph has no negative frequencies, and any "calculated" negative frequencies become positive frequencies via the Trig identity: cos(−ωit) = cos(ωit).
We can get around this image problem by working in the domain of Complex Numbers (numbers with a real and an imaginary component). Specifically, we will use exponentials with complex arguments.
Complex Exponentials -- A Quick Review of Some Important Identities:
Euler's Formula:
Similarly,
From Euler's Formula it can be shown that:
Frequency Conversion using Complex Numbers in Exponential Form:
Let's use the above equations to shift signal frequencies.
First, for ease of demonstration, let's define an input RF signal, x(t), to be a simple cosine at a single frequency, fi Hz. I.e.:
To minimize my typing, I will express this signal's frequency as its radian equivalent, where ωi = 2πfi.
Using the identities above we can express cos(ωit) as the sum of two complex exponential numbers:
Now let's now define our oscillator's signal as a complex exponential:
(Note that from Euler's Formula ejωot = cos(ωot) + jsin(ωot)).
Our next step is to multiply together the input signal and the oscillator signal. We will call the output y(t).
Replacing the input signal with its equivalent complex exponential form, the output equation becomes:
Finally, let's finish the multiplying. The result is:
Note that the frequency components in the arguments of both exponentials have been shift up by ωo.
By the way, we can use Euler's Formula to express this result in terms of sines and cosines:
You can also derive the same result using only sines, cosines, and trigonometric identities and not complex exponentials, but the math is more cumbersome.
OK, so an NCO's output that is a complex-exponential with an argument whose frequency is +ωo will shift the incoming signal's frequency up by ωo. What happens if the NCO's frequency is negative, i.e. -ωo?
Using the same approach as above, the DDC's output can be shown to be:
Again, note that y(t) can be expressed as the sum of sines and cosines. Using the identities at the start of this post:
which is exactly equivalent to y(t) = (ej(ωi−ωo)t + ej(−ωi−ωo)t)/2.
Mixing with Complex Exponentials:
With a view towards understanding SDR building blocks, let's look at a Digital Mixer using the down-conversion math I've just discussed, and which shifts signal frequencies in the digital (rather than analog) realm.
Below is a Digital Mixer block diagram using complex numbers. Let's drive the RF input with a simple cosine signal, cos(ωot):
The Digital Down Converter (DDC) is a basic block of FPGA-implemented SDR radios. Per Wikipedia:
A digital down-converter (DDC) converts a digitized, band limited signal to a lower frequency signal at a lower sampling rate in order to simplify the subsequent radio stages.Below is an illustration of a DDC stage (don't worry about the math -- it will be explained later in this post):
(Click on image to enlarge)
Note that, for demonstration purposes, the equations assume a simple cosine as the input signal.
This post will look at the math involved in the Mixer. I will leave the low-pass filter and sample-rate conversion for later posts.
First, some basics...
Basic Down-Conversion Mixing:
Historically, down-conversion has been done in the analog domain with a "real" oscillator signal (e.g. cos(ωot)). Let's first look at this type of basic mixer before tackling mixing in the Digital (and Complex) domain.
For demonstration purposes, I'll simplify the receive-signal to be a just simple cosine signal at a single frequency: cos(ωit).
This mixer's math is straightforward. Let the output of the mixer be y(t).
y(t) = cos(ωit)*cos(ωot)
We can use Trigonometric identities to express this equation in terms of
sums and differences of frequencies:
y(t) = (cos((ωi−ωo)t))/2 +
(cos((ωi+ωo)t))/2
This type of conversion is known as "Direct Conversion." For an example, see Rick Campbell's R1 receiver.
Visually, here's how the frequencies shift, shown as a single-sided spectrum (which one would see if one did a "Trigonometric" Fourier Transform on the RF input. Refer to equation 27 in this paper by Robert Marcus for more detail)::
Note the overlap of the two signals down at base-band...our "desired" signal would be interfered with by any signal at its "image" on the other-side of the oscillator's frequency!
And if we were to consider the Mixer's input to be a band of frequencies:
You can see the spectrum fold-back with the result being the desired signal and its undesired image occupying the same base-band frequencies. A single-sided spectrum graph has no negative frequencies, and any "calculated" negative frequencies become positive frequencies via the Trig identity: cos(−ωit) = cos(ωit).
We can get around this image problem by working in the domain of Complex Numbers (numbers with a real and an imaginary component). Specifically, we will use exponentials with complex arguments.
Complex Exponentials -- A Quick Review of Some Important Identities:
Euler's Formula:
ejθ = cos(θ) + jsin(θ)
Similarly,
e−jθ = cos(θ) - jsin(θ)
cos(θ) = (ejθ + e−jθ)/2
sin(θ) = j(e−jθ − ejθ)/2
Let's consider a real signal at a single
frequency fo (Hz), and let's express this signal
simply as a cosine: cos(2πfot).
Using the identities above this signal can be expressed in exponential form:
Below is a representation, in the complex frequency-domain, of this signal
:
Using the identities above this signal can be expressed in exponential form:
cos(2πfot) = (ej2πfot +
e−j2πfot)/2
And, for completeness, here are a two more illustrations:
First, to complement the cosine representation above, here is a complex frequency-domain representation of a sine wave signal of the same frequency:
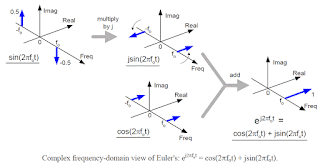
Second, we can use both of these illustrations of the signals in the complex frequency-domain to generate a visual representation of Euler's Formula, showing how the imaginary sine terms are rotated (when multiplied by j) to the real axis and then added to the real cosine terms:
Note that multiplying sin(2πfot) by j rotates the sine's complex frequency-domain imaginary components "clockwise" by 90 degrees to the real plane. If we were to instead multiply sin(2πfot) by -j, the rotation would be 90 degrees counter-clockwise, with the results again being on the real plane, but pointing in the opposite direction, and our final result (after adding the real components together) would represent Euler's Formula for a negative frequency, -fo, instead of fo.
First, to complement the cosine representation above, here is a complex frequency-domain representation of a sine wave signal of the same frequency:
Second, we can use both of these illustrations of the signals in the complex frequency-domain to generate a visual representation of Euler's Formula, showing how the imaginary sine terms are rotated (when multiplied by j) to the real axis and then added to the real cosine terms:
(Click on image to enlarge)
Note that multiplying sin(2πfot) by j rotates the sine's complex frequency-domain imaginary components "clockwise" by 90 degrees to the real plane. If we were to instead multiply sin(2πfot) by -j, the rotation would be 90 degrees counter-clockwise, with the results again being on the real plane, but pointing in the opposite direction, and our final result (after adding the real components together) would represent Euler's Formula for a negative frequency, -fo, instead of fo.
Let's use the above equations to shift signal frequencies.
First, for ease of demonstration, let's define an input RF signal, x(t), to be a simple cosine at a single frequency, fi Hz. I.e.:
x(t) = cos(2πfit)
To minimize my typing, I will express this signal's frequency as its radian equivalent, where ωi = 2πfi.
x(t) = cos(ωit)
Using the identities above we can express cos(ωit) as the sum of two complex exponential numbers:
cos(ωit) = (ejωit +
e−jωit)/2
Now let's now define our oscillator's signal as a complex exponential:
ejωot
(Note that from Euler's Formula ejωot = cos(ωot) + jsin(ωot)).
Our next step is to multiply together the input signal and the oscillator signal. We will call the output y(t).
y(t) = cos(ωit) * ejωot
Replacing the input signal with its equivalent complex exponential form, the output equation becomes:
y(t) = [(ejωit +
e−jωit)/2]*ejωot
Finally, let's finish the multiplying. The result is:
y(t) = (ej(ωi+ωo)t +
ej(−ωi+ωo)t)/2
Note that the frequency components in the arguments of both exponentials have been shift up by ωo.
By the way, we can use Euler's Formula to express this result in terms of sines and cosines:
y(t) = [cos((ωi+ωo)t) + jsin((ωi+ωo)t)]/2 + [cos((-ωi+ωo)t) + jsin((-ωi+ωo)t)]/2
You can also derive the same result using only sines, cosines, and trigonometric identities and not complex exponentials, but the math is more cumbersome.
OK, so an NCO's output that is a complex-exponential with an argument whose frequency is +ωo will shift the incoming signal's frequency up by ωo. What happens if the NCO's frequency is negative, i.e. -ωo?
Using the same approach as above, the DDC's output can be shown to be:
y(t) = (ej(ωi−ωo)t +
ej(−ωi−ωo)t)/2
In other words, both of the input signal's complex exponentials are shifted
down in frequency by ωo. Voila, a Digital Mixer!Again, note that y(t) can be expressed as the sum of sines and cosines. Using the identities at the start of this post:
y(t) = [cos((ωi-ωo)t) + jsin((ωi-ωo)t)]/2 + [cos((ωi+ωo)t) - jsin((ωi+ωo)t)]/2
Mixing with Complex Exponentials:
With a view towards understanding SDR building blocks, let's look at a Digital Mixer using the down-conversion math I've just discussed, and which shifts signal frequencies in the digital (rather than analog) realm.
Below is a Digital Mixer block diagram using complex numbers. Let's drive the RF input with a simple cosine signal, cos(ωot):
Note that the oscillator's output is a complex exponential with a negative frequency in its argument. This negative frequency, as I've discussed earlier in this post, will down-convert incoming RF.
The input signals' spectrum (shown below as two-sided, with negative frequencies, per the frequencies in the complex arguments in the exponential form of the input signal) will shift down:
(Click on image to enlarge)
The illustration below shows a more general case for a band of frequencies at the DDC's input:
(Click on image to enlarge)
Note that, unlike the analog mixer described at the start of this post, this spectrum has both positive and negative frequencies. In other words, we do not (yet) have an issue with "image wrapping" while we remain in the complex domain.
Another take on the same topic, from "Complex Signal Processing is Not -- Complex," by Ken Martin, showing the same:
(Source: "Complex Signal Processing is Not -- Complex,",Ken Martin)
Note (again) that the input consists of only real, not complex, numbers, but the output Y(t) will be in complex form, with both real and imaginary components.
Regarding the mixer (c) in the image above, we can express its two outputs (
First, for simplicity of analysis let's define the input xr(t) to be a simple cosine at frequency ωr:
x(t) = cos(ωrt)
Noting that the oscillator frequency is ωi in this example, the output yr(t) is therefore:
which, using trig identities, expands to:
yr(t) = cos((ωr−ωi)t)/2 +
cos((ωr+ωi)t)/2
Now let's do the same to derive yq(t), the imaginary term...noting that −sin(ωit) = sin(−ωit), yq(t) becomes:
yq(t) =
sin((ωr−ωi)t)/2 − sin((ωr+ωi)t)/2
Is this result equivalent to the result derived (above) using complex-exponential equations?
Essentially, yes it is!
Let's multiply the yq(t) channel by j (i.e. add a phase shift of 90 degrees with, say, a Hilbert Transform) and call this output yq(t)' (note the 'prime'). Its equation is:
yq(t)' = jsin((ωr−ωi)t)/2 −
jsin((ωr+ωi)t)/2
Which, from Euler's Formula, we know is exactly equivalent to:
and which is exactly equivalent in form to the equation we derived earlier:
y(t) = (ej(ωi−ωo)t +
ej(−ωi−ωo)t)/2
Links and Other Resources:
An Excellent Tutorial on Complex Numbers: Quadrature Signals: Complex, but not Complicated, Richard Lyons
An interesting site to surf:
https://www.dsprelated.com/freebooks/mdft/Positive_Negative_Frequencies.html
An interesting site to surf:
https://www.dsprelated.com/freebooks/mdft/Positive_Negative_Frequencies.html
Representing a signal as the sum of two exponentials: http://ece.mst.edu/media/academic/ece/documents/classexp/ee216labs/EE216_Lab4.pdf
The Significance of Negative Frequencies in Spectrum Analysis, Robert Marcus, IEEE Transactions on Electromagnetic Compatibility, Dec. 1967. (A copy can be found here).
Good tutorial on Complex Number in Radio applications: Complex Signal Processing is Not -- Complex, Ken Martin
Standard Caveat:
I could have easily made a mistake in any of the above text, equations, or
drawings. If something looks wrong or is confusing, please feel free
to contact me. Thanks!























2 comments:
Great job congratulations!
73s
LU2JJF
Outstanding work. <3
Post a Comment